This puzzle is a toroidal Gokigen-Naname puzzle; in order to adapt the puzzle type, the rules are slightly tweaked, as follows:
Draw a slanted line in each dotted-line square.
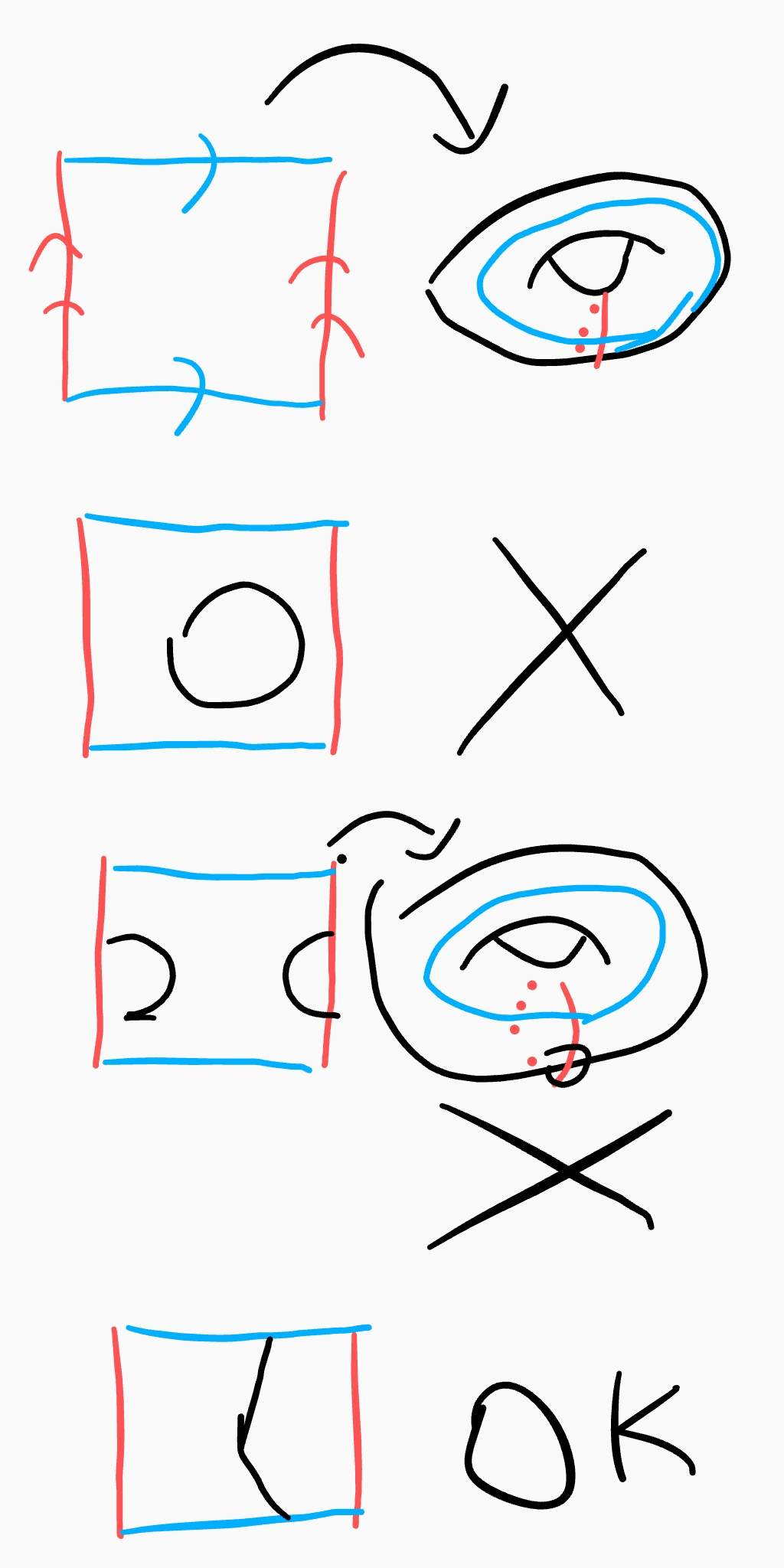
The red and blue lines in the Penpa link and below image are connected as in the standard presentation of the torus, as one might infer from the repeated numbers on each edge.
Treating those numbers on the corresponding location of a line as the same number and adding up the lines entering them, the number of lines entering each circled number should be exactly that number.
The rule forbidding loops has to be relaxed:
If the puzzle is tessellated along the red and blue lines (as in a tiled wallpaper, or the “parallel universes” of Super Mario 64), it should contain no loops.
Equivalently, if you’re familiar with topology, there should be no loops that are trivial in the sense of the fundamental group.
Equivalently, there should be no loop separating the inside and outside.
Furthermore, an image describing allowed versus disallowed loops has been attached to the page, below the image post.
This puzzle was entered into a Logic Showcase on the Puzzlers Club Discord server; it got a tie for 5th place (described by the organizer as “5th and 6th place” with a single vote score), which is a pretty solid result given that there were over 20 entries!
There are two links on which you can play; the penpa link has a proper answer check, but as the “diagonal” line interface can be less intuitive than that of the puzz.link Slant player, I also provide a link to the latter.